Metaphysics & Spirituality _|_ Issue 14, 2020
Wholeness, Timelessness and Unfolding Meaning
An interview from the archives with physicist and philosopher David Bohm


Wholeness, Timelessness and Unfolding Meaning
An interview from the archives with physicist and philosopher David Bohm
This conversation between Professor David Bohm and two editors of Beshara Magazine, Jane Clark and Michael Cohen, took place in August 1990, just two years before his death. It never appeared, as the original Beshara Magazine ceased publication early in 1991. Returning to it now, it is a pleasure to be reminded of the scope and continuing relevance of this remarkable man, who was without doubt one of the most original thinkers of our time. Here he talks about his insight into the essential unbroken wholeness of the universe: the timeless order which lies behind physical phenomena, and the importance of the imagination for giving a meaningful understanding of reality.
 David Bohm (1917–1992) has been described as one of the most significant thinkers of the twentieth century. A theoretical physicist whose radical theories challenged the standard interpretation of quantum mechanics, his interests and influence extended far beyond the narrow confines of science. He worked on theories of mind and consciousness, and had close relationships with the Indian teacher Jiddu Krishnamurti [/] [1] and, later in life, the Dalai Lama, who called him his ‘science guru’. Finding Cartesian duality limited, he believed that the same principles which underlie the behaviour of matter also operate in the realms of consciousness, society and culture. At its deepest level, he maintained, reality is an ‘unbroken whole’, and he made this the ground of his work in every sphere.
David Bohm (1917–1992) has been described as one of the most significant thinkers of the twentieth century. A theoretical physicist whose radical theories challenged the standard interpretation of quantum mechanics, his interests and influence extended far beyond the narrow confines of science. He worked on theories of mind and consciousness, and had close relationships with the Indian teacher Jiddu Krishnamurti [/] [1] and, later in life, the Dalai Lama, who called him his ‘science guru’. Finding Cartesian duality limited, he believed that the same principles which underlie the behaviour of matter also operate in the realms of consciousness, society and culture. At its deepest level, he maintained, reality is an ‘unbroken whole’, and he made this the ground of his work in every sphere.
In Bohm’s early scientific career he worked alongside people like Robert Oppenheimer, Albert Einstein, John Wheeler and Richard Feynman at UC Berkeley and Princeton, making significant contributions to quantum and plasma theory. He came to feel, however, that the Copenhagen Interpretation [/] of quantum mechanics was incomplete and, encouraged by Einstein, began to develop an alternative ‘hidden variables theory’ [/], which he set out in a paper in 1952 [2]. This proposed that the famous indeterminacy of quantum phenomena – that it is not possible to measure the position and the velocity of a particle simultaneously – could be explained by factors operating causally at an unobservable level.
Bohm continued to develop his theory after he left the USA, first in Brazil and then in the UK. In 1980 he published his seminal work Wholeness and the Implicate Order [3] in which he suggested that all the phenomena that appear in the world – whether they be fundamental particles or thoughts in the mind – emerge out of a deeper order of reality, their character varying according to the context (see the video right or below for a good exposition on this). In his final years, he worked with his colleague at Birkbeck College in London, Basil Hiley, to put mathematical flesh on the bones of his insights, and their joint work The Undivided Universe [4] was published posthumously in 1993.
Video: Art Meets Science and Spirituality in a Global Economy, 1990. Duration 7:32 minutes
Alongside this major work, Bohm made many important contributions to physics. His work on quantum vectors, for instance, with one of his students, known as the Aharonov–Bohm Effect [/], was considered by many to be worthy of a Nobel Prize in its own right. But his fundamental reformulation of quantum theory has not been widely embraced by the physics community, although there has been renewed interest recently with the appearance of experimental evidence [/] [5] which validates his approach. This might yet re-establish him within the mainstream. In addition, this spring will see the release of a documentary, Infinite Potential, which includes, along with contemporary physicists, tributes from the Dalai Lama, artist Antony Gormley and native elder Dr Leroy Little Bear attesting to his wider cultural influence.
This interview with Jane Clark and Michael Cohen took place in August 1990 at Bohm’s office in Birkbeck College, but it was never published. Coming back to it after thirty years, it is remarkable to see how fresh and relevant the ideas are, despite the huge developments which have taken place in science. Some things are perhaps even more relevant – his critique of the role of mathematics, for instance, given the way that algorithms have gained control over so many areas of our contemporary lives, and his insight that physical laws may be subject to change and evolution.
A letter sent by Albert Einstein to David Bohm when he was at Sao Paolo University and seeking a position in Israel. In 2017 it was sold by Winners in Israel for $84,000. Click image to enlarge
Unbroken Wholeness
Jane: Can we begin by talking about the idea of unity, which is the central principle with which Beshara Magazine is concerned. My understanding of your work is that you start with the concept of unity – wholeness – and explain how particular phenomena emerge from it, rather than deriving unity from the parts. Can you say something about how you came up with this idea?
David: If we go back to Newtonian concepts, Descartes, etc., the whole idea of physics was analysis into separate parts which are eternally related. That worked quite well for a long time. But in more modern physics, like relativity and quantum theory, it is clear that if you attempt to define these point particles, you cannot do it in the classical way; we cannot regard them as independent separate things, like billiard balls, which interact with each other only in the exterior.
One idea for getting around the problem in relativity is to try and make them into extended points, but there is no consistent way of doing that because the theory dictates that they cannot be rigid. The other idea is to imagine them as dimensionless points, but that leads to other problems. Therefore it is not possible to conceive of the whole as being made out of a collection of separate parts in Einstein’s theory; the idea of unbroken wholeness is implied.
With quantum mechanics, there are all sorts of new properties which do not cohere very well with relativity, but lead you to unbroken wholeness in another way. You have the notion of quantum jumps of energy that are said to be discrete and unanalysable – in other words, the basic energy comes in the form of quanta – and the idea is that everything is connected by those. Therefore in the final analysis, it is again all one unbroken whole.
Secondly, there is the phenomenon of wave-particle duality: that an electron going through two slits behaves like a wave, and yet it arrives at the detector like a particle. So it appears to have a nature that depends upon its context, and this again suggests that the particle does not have its own separate nature entirely, but is internally related to the whole, or at least to its environment. And a third thing is quantum non-locality, in which under certain conditions you can find a close connection between things which are physically distant. This violates classical ideas, and provides another way in which a system of particles unites into a whole which has an objective feature of wholeness, meaning that it is not reducible to actions of parts.
It was clear to me that there are problems in bringing relativity and quantum theory together because relativity demands strict causality, strict continuity and strict locality, whereas in quantum mechanics the behaviour of individual particles is basically unpredictable, and there are also these issues of discontinuity and non-locality. So the basic concepts of the two theories do not cohere, although there are certain mathematical algorithms and experiments that make them work together. Therefore, it seemed to me that in order to bring them together you would have to say that the common ground is undivided wholeness.
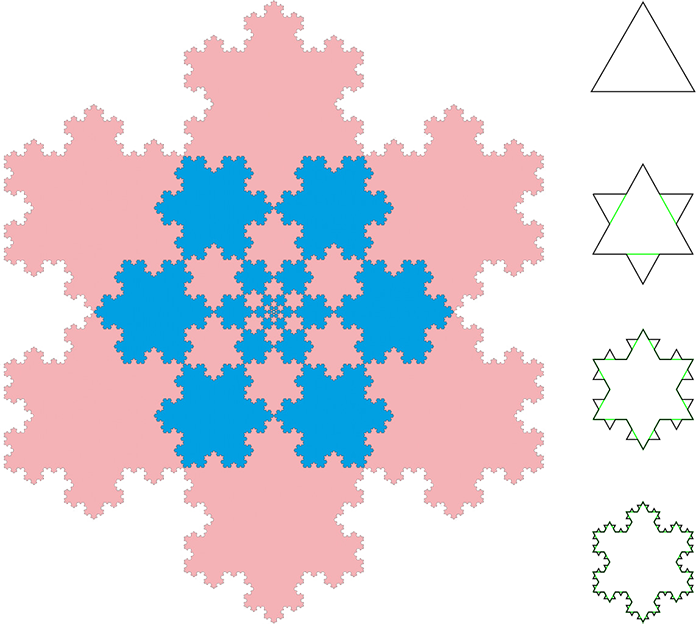
Bohm saw fractals as a vivid example of what he called ‘a generative order’. This is the Koch Snowflake Fractal [/], discovered by the Swedish mathematician Helge von Koch [/] in 1904, which is generated from a simple equilateral triangle. Images via Wikimedia Commons
The Implicate Order
.
Jane: So in your view, all the things that we see in the world might look as though they are separate and independent of each other, but they are actually manifestations of a deeper reality. What we see is like the tip of an iceberg.
David: In my theory of the implicate order, the whole generates the particulars, rather than saying that the particulars are collected to make the whole. We must be aware, however, that our concept of the whole is always going to be limited, so when we are thinking about what the implicate order is, we need to open up our ideas about it and consider it as a particular again. So we need a two-way movement between the general and the particular. The root meaning of general is that it is generated, so from that point of view, we can say that the whole generates the particulars, but it is also generated.
Michael: If the particulars emerge out of the generative background, then they must always have been implicit within it?
David: Yes. They emerge from it, and then they develop and so on, and eventually they are enfolded back into it. The background – the general, the implicate order, however you want to speak about it – is basically unlimited, so one cannot capture it finally and define it. People refer to it in terms like ‘ineffable’.
Jane: What we tend to do is to extrapolate from what we know to what we don’t know. But you point out that what we call ‘the general’ or ‘the whole’ is not just a larger version of what we see: it has a different nature.
David: It is a dynamic process of particularising and creating the particulars. But it is important to realise that there is no real division between the implicate and the explicate. The undefinable creates the definable, and that in turn, falls back into the undefinable. The terms ‘definable’ and ‘undefinable’ are both abstractions, and the ultimate generative process is between them.
But at the same time, the explicit is within the implicit and not the other way round. We can see this in vision. We have the vision from out of the corner of the eye, which is vague but catches movement very nicely, and we have vision in the centre which is very sharp. If the centre is damaged, you can still use the other part, but if the periphery is damaged, then the centre has no meaning. So this kind of thing also holds for the mind; there is the generative background emerging into the well-defined focus, on which we may concentrate our attention. But we also have to give our attention to the general, to the generator, and this means developing undirected attention.
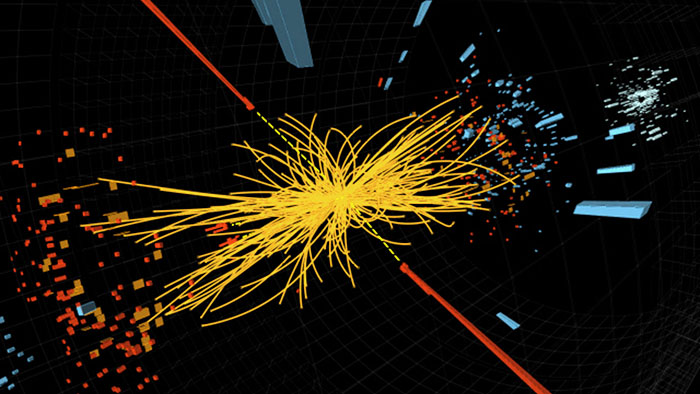
Proton–proton collision event in the Great Hadron Collider at CERN, in which four high energy electrons (green lines and red towers) are observed. According to Bohm, the behaviour of these fundamental particles will only be properly understood when they are seen as emerging from a deeper (implicate) order of reality. Photograph: Thomas McCauley, Lucas Taylor, CMS, © 2011 CERN
Infinite Subtlety
.
Jane: So you think that whilst the whole may be undefinable and unknown, there are nevertheless ways in which we can have access to it?
David: One of the things I want to distinguish in all my work is the difference between the subtle and the manifest. The manifest is what can be held in the hand, in the eye or in the head; this is the explicate order. The other side of this is the subtle. To define something means to ‘grasp’ it, so that which cannot be grasped is undefinable, and whatever is beyond such limits has to be subtle. Infinity does not really mean more and more space, or more and more time – these are rather crude conceptions of it – but rather, it means more and more subtlety. The nature of the implicate order is that it is subtle, and within it there are many different levels of subtlety. These deeper things could be like vibrations that we can sense, as we might sense more and more subtle feelings, pointing to something out of which ideas and images emerge.
Take for example all the experience of ourselves that we have at the ordinary level, in which I include all the various kinds of thoughts, images and sensations with which we identify. Now ordinarily we say, tacitly, that that is me. But that is really only an explicit image of something much more subtle and enfolded. If you take that image as what is real – seeing it as independent – then things don’t work because it is as if you are looking at a mirror image. In using this analogy, I don’t mean that there is a one-to-one correspondence between forms at the implicate and explicate levels: it is more complex than that.
When you look at yourself in the mirror and try to shave, at first you cannot do it because things are the wrong way round. So you have to learn how to deal with a mirror image. I think that the mind has the property of being able to what I call ‘search’ into these implicate levels; maybe you could compare this to millions of fingers feeling outward, searching into the depths of the implicit. Then when it finds something close to what it wants, it homes in on it.
When we look at what we call ourselves, we are looking at a rather explicate image of something which is very, very different, and there has to be a searching movement to feel out what is happening. Then the image becomes valuable as a reflection of the deeper reality. But otherwise, the image can become a source of confusion and we begin to value it too highly; it becomes ego, and we have worship of the self-image, etc.
I think that science can be looked at in this way too. We get all sorts of explicit images – things that happen – but we have to look deeper to find out what underlies them. If you try to deal directly with them at their own level, then things become incoherent. You can do it up to a point, but the method is limited.
Michael: A good example of this might be the way that fundamental particles seem to fleetingly appear and disappear during high speed collisions in particle accelerators.
David: Yes, but the same can be said more fundamentally about our notions of space and time. That whole order has to be seen as limited; it does not stand by itself. We have a tacit thought that everything that exists exists in time and space, and this is very deep in our culture. But this may not cohere with what we are finding now in physics and many other areas.

Bohm in conversation with Jiddu Krishnamurti at Brockwood Park in Hampshire, UK. Some of their many dialogues [/] were published as The Ending of Time in 1985 [1]. Photograph: Mark Edwards © Krishnamurti Foundation Trust
The Problem of Time
.
Jane: The question of time seems particularly relevant at the moment, and is a particular source of confusion.
David: One point about any theory that people develop about time is that it always presumes some timeless principles, and without these it would not really have anything to say. So in some way, people cannot escape from the question of what is timeless; they merely put it aside and get on with their work on time. You can make progress in this way, but there is a limit to how far you can get.
Time is a mystery from a scientific point of view. The past is gone and the future has not yet happened, and the present as the point between past and future immediately ceases to exist – or it is possible to question whether it ever existed at all. Therefore some people have said – and Einstein was one of them – that only this block of time/space is real, and they do not engage with the question of timelessness.
But then you get into trouble when you start to deal with the experience of process, because we say that here we are moving through time, and we propose that the relationship between what is and what was is velocity. But how can you make a relationship with something that has already gone, which is not? And how can the basics of necessity be found in such a relationship? The problem with thought is that it always has to make a static abstraction in order to explain things, so it will never be able to grasp process totally, although it may give some insight, some way of looking at it.
The central problem, I think, is that we put necessity in the order of time, and this is what scientific laws are meant to express.
Jane: Can you say a bit more about what you mean by ‘necessity’ here?
David: Necessity means that it cannot be otherwise. Contingency means that it can be otherwise. The division between the two is crucial for any way of thinking – although of course they are not ultimately divided: they are two aspects.
Now, another way of saying this is that necessity is what cannot be turned aside. When we think about natural laws, one of the first things to say is that the word ‘law’ is a very bad one, because it sounds as if someone has legislated the way things are. People used to believe that they were legislated by God but most scientists don’t believe that now; so what do they think is the source of this legislation? In ancient Greek philosophy, they did not talk about laws but about a regular order which appears in nature. This regular order, if it were necessary, would have the same meaning as our word ‘law’.
But as I just pointed out, we cannot really understand time in this way because there is so much paradox. You can say, as Einstein did, that all time is there in every moment – but then there are other problems. For instance, it implies that in some sense our experience of time and movement, and so on, is an illusion, and people have not really managed to formulate anything coherent about this. The nearest they can get to it is to say that our memory is part of the process, and that each successive moment has a separate memory. But still they don’t explain the succession; what is this necessity – this real compulsion – to move from one moment to the next?
So I don’t think the problem can be solved within the framework we have. Necessity is meant to hold for all time – but here we have only one moment. But if we say that all time is there in that one moment, then we have the problem of succession.
Jane: Do you think you can solve this within the framework that you have developed?
David: I don’t think it will be an easy thing to solve. Our theory says that the whole – that is, the implicate order – is an order beyond time, and that time emerges from it. One moment forms, and then another moment, and then another, and so on. And if they form in a similar way, then we have a succession with some similarity – and also, some difference because nothing remains totally constant. The general rules that we use to talk about succession are also grounded in the implicate order, just as the moments themselves are grounded in it. So although they are constant, they are only relatively so. Putting it like this would at least provide a coherent way of talking about time and making our assumptions explicit.
Jane: You mean, assumptions such as the timelessness of the laws that are used?
David: Yes. The important thing here is that the necessity for the time sequence is not in the time sequence itself. This is crucial. People have been looking for it in the wrong place. You can find a kind of relative necessity in the succession: but relative necessity is not really necessity but is more like the Greek idea of a regular order; it is still open to contingency. People like Rupert Sheldrake have brought this out recently.
Michael: You are referring to his theory of morphic resonance (see our recent interview) [6], which maintains that the natural laws themselves may be evolving.
David: Yes. But there is also a problem with this, in that you are making evolution a law, and where is the ground of that? So I think that the way forward is to consider this ground, this wholeness, which is not of the order of time and space. Time emerges from this, but with sufficient regularity that we get the impression of necessity.
Michael: Could it be formulated that necessity and contingency are both in some way present within this timeless level?
David: Yes, we can do this, but with the awareness that this whole thing might itself be contingent – it is just a stage worth exploring.
Jane: Is it not part of current theories of the Big Bang that time emerges from the initial creative impulse?
David: Yes, but it is not clear at all what it arose from. It should be made much more clear that what is implied is a timeless order. Of course, cosmological theories are constantly in flux so our understanding is changing all the time. But the basic philosophical questions about time and necessity remain.
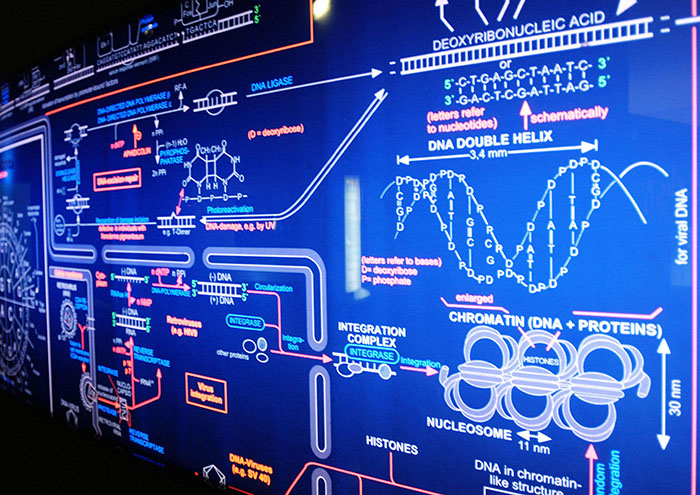
Genetic algorithms, Miramon Kutxaespacio de la Ciencia Museum, San Sebastian, Spain, showing how even research in the life sciences is now employing mathematical computation. Image: agefotostock [/] / Alamy Stock Photo
Mathematics and Imagination
.
Michael: Also remaining are your observations about the way that thought works, and the processes by which we come up with scientific theories?
David: Yes. One of the problems we have these days is the tendency to see mathematics as giving absolute truth. We think that expressing things in mathematical terms makes them more precise, but maths is actually very clumsy in some ways. When you write down an equation, you may be making all sorts of absurd assumptions and it can take you a very long time to realise this. I am not saying that we should not do maths but we need to be aware of what we are doing, and it is the lack of awareness that I object to. It can take a long to time to work out what your equations really mean, and so maths tends to take away the intuitive understanding of things.
Michael: You have talked a lot about the need for intelligibility in science and finding ways of expressing what is really going on in terms of physical process.
David: Yes. This is the distinction between having what I would call ‘mechanical knowledge’ and understanding. You can have an algorithm which calculates everything, but that is not the same as understanding what is really going on. There is a very strong tendency in modern science to believe that one day we will develop a theory of everything and have an algorithm which will do everything. Stephen Hawking [7] was one of the proponents of this; he thought that this will happen in the near future and then theoretical physics will be replaced by computers.
Modern science has developed in a very mathematical direction; James Jeans said in the 1920s that God must be a mathematician, and Heisenberg later said something of the same nature. The imagination has come to be seen as something which unfolds the meaning of the mathematics, whereas in previous centuries imagination was taken as the source of understanding and the mathematics followed on, making the intuitions sharper.
This has happened to such an extent that most physicists nowadays feel that the mathematics are their main contact with the universe. Experiments are seen as a much more tenuous form of contact which are only really there to test the mathematics. So modern physicists feel that all we can really talk about are the equations; whilst imaginative ideas might be useful in understanding them, the equations are what are true, and they are what is revealing the real pattern of the universe.
Michael: But you would not deny that there is a role for mathematical formulations?
David: I think you can also be creative mathematically, but that does not mean that maths gives a complete picture. I myself feel that the intuitive/imaginative content is crucial, because the imagination is basically the connection between the unknown – the unlimited – and the limited. You feel out the unknown and what comes up is expressed through the imagination. Then it becomes more defined through reason, and eventually through mathematics. Scientists still use the imagination in some way because they find very picturesque words to describe the mathematical concepts or the fundamental particles they come up with, but those words are not in any real correspondence with what is going on.

Albert Einstein. Photograph: Informiguel Carreño (own work) via Wikimedia Commons
Jane: From what I have read, Einstein was an example of someone who did use the imagination; he arrived at his great insights through intuition, and only later did he put them into mathematical form.
Bohm: Yes, Einstein was extremely intuitive, and he used to say that he did not think in words but in images – in changing images and recurrent images. He probably thought rather like a very young child, and one of the clues to this is that he did not learn to talk until he was quite old – the age of three or something – so he took that mode of thinking quite far. In most people, the verbal thinking comes in and overwhelms it. Krishnamurti also thought that the word is rather overdone in our culture, and that we have to get to what is behind the words. This is common ground between these two people who might otherwise seem to be at opposite ends of the spectrum.
Kristhnamurti was also not so keen on images; he used a lot of images and metaphors himself, but he felt that we need to get beyond image. It might look, in some of his writings, as though he advocated giving up the imagination altogether, but there were some things that he said that indicated that he thought there was a place for the creative imagination.
Einstein, of course, used the creative imagination extensively. He did not get his main ideas from experiments, but rather from his whole perception and experience of life. Einstein was a mystic in his own way, on the cosmic side of things, and he was deeply interested in that. This is another similarity to Krishnamurti, who was also most interested in the cosmic side of things. He thought that the cosmos was intelligent and compassionate, and he believed that it could speak through the individual.
Jane: By ‘cosmos’, he did not mean just the physical world?
David: No, it went much deeper than that.
Michael: As you have been talking, I have been thinking about aesthetics, and about beauty, which can point to both its own limitedness and to the unknowable ground beyond.
David: Beauty is a very key part of science, and many scientists and mathematicians have talked about it. One thing about beauty is that it involves coherence.
Michael: By which you mean order?
David: Yes. Sustained incoherence cannot be felt as beautiful, although some incoherence can be integrated into a higher level of coherence. So we don’t ask for perfect coherence; creativity demands some level of incoherence. That which is too symmetric and closed is not experienced as truly beautiful.
So beauty can be sensed as coherence, and it can also be perceived in certain ways with the senses. This goes very deep in what, in my terminology, I call the enfolded order: the sensing of beauty – perceiving it through the senses, I mean – probably goes deeper than the intellectual grasp of beauty as coherence. I think that people who are creative have always known this – Einstein and Poincaré and so on. I think in modern times we have become more hard bitten and people are always asking about getting value for money and so on; they don’t want to give things like beauty importance.

Portrait of David Bohm by Mark Edwards. Photograph © Mark Edwards / Hard Rain Project
Image Sources (click to close)
Banner: David Bohm. Photograph © Mark Edwards/Hard Rain Project.
First inset: Cover of Bohm’s highly influential book, Wholeness and the Implicate Order, published in paperback in 1983.
Other Sources (click to open)
[1] DAVID BOHM & J. KRISHNAMURTI: The Ending of Time: 13 Dialogues Between J. Krishnamurti and David Bohm (Gollancz,1985). For more information and to watch some videos, click here [/].
[2] DAVID BOHM: A Suggested Interpretation of the Quantum Theory in Terms of ‘Hidden’ Variables. (Phys. Rev. 85, 166, 1952). Click here [/].
[3] DAVID BOHM: Wholeness and the Implicate Order (1980: Routledge, 2002).
[4] DAVID BOHM & BASIL HILEY: The Undivided Universe (Routledge, 1993).
[5] DYLAN H. MAHLER et al: ‘Experimental nonlocal and surreal Bohmian trajectories’ in Science Advances (19 Feb 2016, Vol. 2, no. 2). Click here [/].
[6] RUPERT SHELDRAKE: A New Science of Life (Blond and Briggs, 1981; 3rd revised edition, Icon, 2009).
[7] STEPHEN HAWKING: A Brief History Of Time: From Big Bang To Black Holes (1988: Bantam, 2011).
Email this page to a friend
FOLLOW AND LIKE US
Video: The Nature of Things. An interview with David Suzuki, 1979. Duration 40:30 minutes
——————————————
——————————————
——————————————
FOLLOW AND LIKE US
If you enjoyed reading this article
Please leave a comment below.
Please also consider making a donation to support the work of Beshara Magazine. The magazine relies entirely on voluntary support. Donations received through this website go towards editorial expenses, eg. image rights, travel expenses, and website maintenance and development costs.
READ MORE IN BESHARA MAGAZINE
Sheldrake: Memory, Habits and Wholeness
Biologist Rupert Sheldrake challenges the dogmas of conventional science, and explains how recent research supports his alternative theory of morphic resonance
Getting Back in Time
Richard Gault argues that technology has radically altered our relationship to time
The Century of Complexity
An interview with Dr. Vasileios Basios on new ideas in science
Connecting with the Unseen World
Kira Perov, wife and long-term collaborator of the video artist Bill Viola, talks to Jane Carroll about the ideas and experiences which inspire their work
READERS’ COMMENTS






Tremendous article. (Referring to parts I seem to understand or feel an intuitive sympathy with.) Such brain power as Bohm’s defending and upholding what might be thought the “sacramental value” of imagination is not as current as it might or should be. Thank you, Beshara!
A beautiful article. So good to have it published at last.
Insightful and much food for thought
thank you
Thank you for publishing this interview now, so many years later, but like so much thought in the last century that has not been accepted is becoming urgently necessary. It comes so well after Andreas Weber’s talk.
Something deep inside me stimulated with joyous tremor and expansiveness.
A delightful article. David Bohm showed in 1952 how to understand a handful of basic experiments in quantum mechanics in a way that was free of mystery, yet predicted all the “strange” quantum mechanical effects that we actually observe in these experiments.
Because his theory worked so well, physicists doubted it and even ignored it. This happened because the standard “Copenhagen Interpretation” of 1927 required us to believe a set of really weird concepts, such as wave/particle duality, probability as the basis of process, and the impossibility of predicting a single observation, when considering physics at scales of the atom or smaller.
These concepts even led some physicists to include the consciousness of the human observer as a basic factor in the observed experimental behavior. Bohm’s simple insight removed the need for either mysticism or human consciousness when explaining the basic experiments. It was the very goal that Einstein and Bell had intuited could be achieved.
Perhaps the ignoring of Bohm’s spectacularly successful theory (along with his concurrent political problems with McCarthy and Oppenheimer) was just the motivation he needed to investigate, working with J. Krishnamurti, the nature of consciousness itself, independent of humans, objects, or any of the usual domains of physics.
Bohm would be so happy to see how things are going today. His theory is beginning to get experimental verification. Those who popularize physics are beginning to explain Bohm’s amazingly simple insights into quantum mechanics, so even physicists are learning about them. And so many nonduality teachers are converging on a consistent understanding of the one consciousness as reality, while at the same time pointing clearly to a simple individual human path to reflecting that one consciousness fully and lovingly.